Primův algoritmus

V tomto kurzu se dozvíte, jak funguje Primův algoritmus. Také najdete pracovní příklady Primova algoritmu v C, C ++, Java a Python.

Primův algoritmus je algoritmus minimálního kostry, který bere graf jako vstup a najde podmnožinu okrajů tohoto grafu, který

tvoří strom, který zahrnuje každý vrchol
má minimální součet hmotností mezi všemi stromy, které lze vytvořit z grafu

Jak funguje Primův algoritmus

Spadá pod třídu algoritmů zvaných chamtivé algoritmy, které nacházejí místní optimum v naději, že najdou globální optimum.

Začínáme od jednoho vrcholu a neustále přidáváme hrany s nejnižší hmotností, dokud nedosáhneme svého cíle.

Kroky pro implementaci Primova algoritmu jsou následující:

Inicializujte minimální kostru s náhodně vybraným vrcholem.
Najděte všechny hrany, které spojují strom s novými vrcholy, najděte minimum a přidejte jej do stromu
Opakujte krok 2, dokud nezískáme minimální kostru

Příklad Primova algoritmu

Začněte s váženým grafem

Vyberte vrchol

Vyberte nejkratší hranu z tohoto vrcholu a přidejte ji

Vyberte nejbližší vrchol, který ještě není v řešení

Vyberte nejbližší okraj, který ještě není v řešení, pokud existuje více možností, náhodně vyberte jednu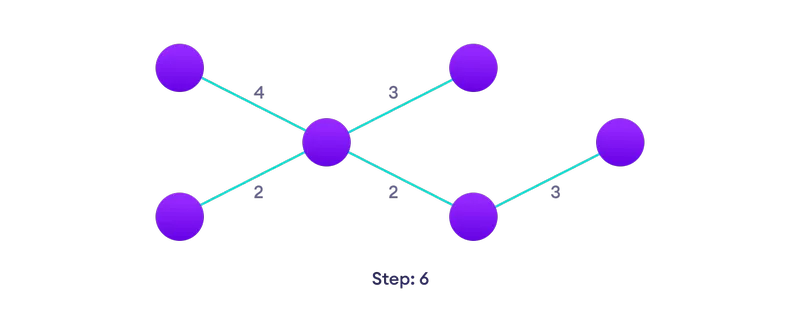

Opakujte, dokud mít klenutý strom

Primův algoritmus pseudokód

Pseudokód pro primův algoritmus ukazuje, jak vytvoříme dvě sady vrcholů U a VU. U obsahuje seznam vrcholů, které byly navštíveny, a VU seznam vrcholů, které nebyly navštíveny. Jeden po druhém přesuneme vrcholy ze sady VU na sadu U připojením hrany s nejmenší hmotností.

 T = ∅; U = ( 1 ); while (U ≠ V) let (u, v) be the lowest cost edge such that u ∈ U and v ∈ V - U; T = T ∪ ((u, v)) U = U ∪ (v)

Python, Java a C / C ++ příklady

I když se používá reprezentace grafů adjacency matrix, lze tento algoritmus také implementovat pomocí Adjacency List pro zlepšení jeho efektivity.

Python Java C C ++

 # Prim's Algorithm in Python INF = 9999999 # number of vertices in graph V = 5 # create a 2d array of size 5x5 # for adjacency matrix to represent graph G = ((0, 9, 75, 0, 0), (9, 0, 95, 19, 42), (75, 95, 0, 51, 66), (0, 19, 51, 0, 31), (0, 42, 66, 31, 0)) # create a array to track selected vertex # selected will become true otherwise false selected = (0, 0, 0, 0, 0) # set number of edge to 0 no_edge = 0 # the number of egde in minimum spanning tree will be # always less than(V - 1), where V is number of vertices in # graph # choose 0th vertex and make it true selected(0) = True # print for edge and weight print("Edge : Weight") while (no_edge  G(i)(j): minimum = G(i)(j) x = i y = j print(str(x) + "-" + str(y) + ":" + str(G(x)(y))) selected(y) = True no_edge += 1

 // Prim's Algorithm in Java import java.util.Arrays; class PGraph ( public void Prim(int G()(), int V) ( int INF = 9999999; int no_edge; // number of edge // create a array to track selected vertex // selected will become true otherwise false boolean() selected = new boolean(V); // set selected false initially Arrays.fill(selected, false); // set number of edge to 0 no_edge = 0; // the number of egde in minimum spanning tree will be // always less than (V -1), where V is number of vertices in // graph // choose 0th vertex and make it true selected(0) = true; // print for edge and weight System.out.println("Edge : Weight"); while (no_edge < V - 1) ( // For every vertex in the set S, find the all adjacent vertices // , calculate the distance from the vertex selected at step 1. // if the vertex is already in the set S, discard it otherwise // choose another vertex nearest to selected vertex at step 1. int min = INF; int x = 0; // row number int y = 0; // col number for (int i = 0; i < V; i++) ( if (selected(i) == true) ( for (int j = 0; j G(i)(j)) ( min = G(i)(j); x = i; y = j; ) ) ) ) ) System.out.println(x + " - " + y + " : " + G(x)(y)); selected(y) = true; no_edge++; ) ) public static void main(String() args) ( PGraph g = new PGraph(); // number of vertices in grapj int V = 5; // create a 2d array of size 5x5 // for adjacency matrix to represent graph int()() G = ( ( 0, 9, 75, 0, 0 ), ( 9, 0, 95, 19, 42 ), ( 75, 95, 0, 51, 66 ), ( 0, 19, 51, 0, 31 ), ( 0, 42, 66, 31, 0 ) ); g.Prim(G, V); ) )

 // Prim's Algorithm in C #include #include #define INF 9999999 // number of vertices in graph #define V 5 // create a 2d array of size 5x5 //for adjacency matrix to represent graph int G(V)(V) = ( (0, 9, 75, 0, 0), (9, 0, 95, 19, 42), (75, 95, 0, 51, 66), (0, 19, 51, 0, 31), (0, 42, 66, 31, 0)); int main() ( int no_edge; // number of edge // create a array to track selected vertex // selected will become true otherwise false int selected(V); // set selected false initially memset(selected, false, sizeof(selected)); // set number of edge to 0 no_edge = 0; // the number of egde in minimum spanning tree will be // always less than (V -1), where V is number of vertices in //graph // choose 0th vertex and make it true selected(0) = true; int x; // row number int y; // col number // print for edge and weight printf("Edge : Weight"); while (no_edge < V - 1) ( //For every vertex in the set S, find the all adjacent vertices // , calculate the distance from the vertex selected at step 1. // if the vertex is already in the set S, discard it otherwise //choose another vertex nearest to selected vertex at step 1. int min = INF; x = 0; y = 0; for (int i = 0; i < V; i++) ( if (selected(i)) ( for (int j = 0; j G(i)(j)) ( min = G(i)(j); x = i; y = j; ) ) ) ) ) printf("%d - %d : %d", x, y, G(x)(y)); selected(y) = true; no_edge++; ) return 0; )

 // Prim's Algorithm in C++ #include #include using namespace std; #define INF 9999999 // number of vertices in grapj #define V 5 // create a 2d array of size 5x5 //for adjacency matrix to represent graph int G(V)(V) = ( (0, 9, 75, 0, 0), (9, 0, 95, 19, 42), (75, 95, 0, 51, 66), (0, 19, 51, 0, 31), (0, 42, 66, 31, 0)); int main() ( int no_edge; // number of edge // create a array to track selected vertex // selected will become true otherwise false int selected(V); // set selected false initially memset(selected, false, sizeof(selected)); // set number of edge to 0 no_edge = 0; // the number of egde in minimum spanning tree will be // always less than (V -1), where V is number of vertices in //graph // choose 0th vertex and make it true selected(0) = true; int x; // row number int y; // col number // print for edge and weight cout << "Edge" << " : " << "Weight"; cout << endl; while (no_edge < V - 1) ( //For every vertex in the set S, find the all adjacent vertices // , calculate the distance from the vertex selected at step 1. // if the vertex is already in the set S, discard it otherwise //choose another vertex nearest to selected vertex at step 1. int min = INF; x = 0; y = 0; for (int i = 0; i < V; i++) ( if (selected(i)) ( for (int j = 0; j G(i)(j)) ( min = G(i)(j); x = i; y = j; ) ) ) ) ) cout << x << " - " << y << " : " << G(x)(y); cout << endl; selected(y) = true; no_edge++; ) return 0; )

Primův vs Kruskalův algoritmus

Kruskalův algoritmus je další populární algoritmus minimálního rozpětí stromu, který používá jinou logiku k nalezení MST grafu. Místo toho, aby Kruskalův algoritmus vycházel z vrcholu, třídí všechny hrany od nízké hmotnosti po vysoké a stále přidává nejnižší hrany, přičemž ignoruje hrany, které vytvářejí cyklus.